 Interferometric Optics Interferometric OpticsResearch on optics, laser optics, and laser physics |
|---|
 Interferometric Optics Interferometric OpticsResearch on optics, laser optics, and laser physics |
|---|
This is a brief tutorial on prism and multiple-prism pulse compression, and compressors, provided from a historical perspective. Prismatic pulse compressors for femtosecond, and ultrafast tunable lasers, were first introduced, via a single-prism configuration, in 1983 by Dietel et al. [1]. Multiple-prism pulse compressor arrays followed in 1984 [2]. Independently, the generalized multiple-prism dispersion theory applicable to prismatic pulse compression was introduced by Duarte and Piper in 1982 [3] and then extended to second order derivatives in 1987 [4]. A double-prism pulse compressor in a femtosecond laser cavity is depicted in Fig. 1.
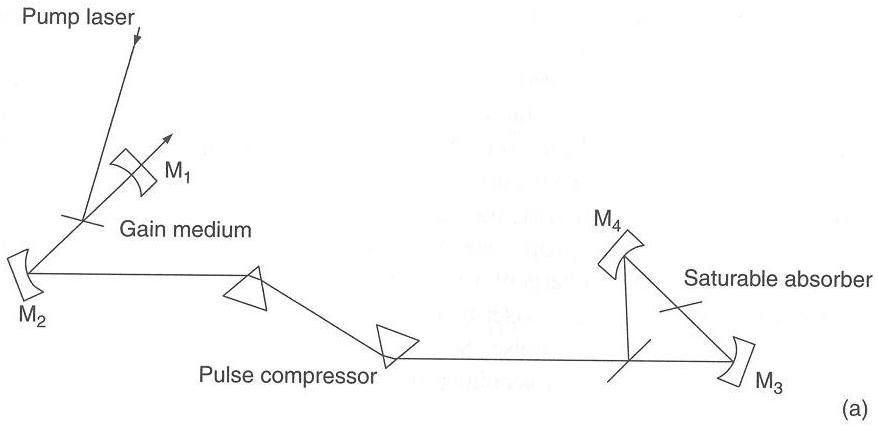
The principles of prism, and multiple-prism, pulse compression are described and discussed by Duarte [5] and Diels and Rudolph [6]. Briefly: to a first approximation the linewidth, or bandwidth, of tunable laser emission is inversely proportional to the dispersion provided by the intracavity elements. Large dispersion leads to narrow linewidth emission. Very small overall dispersion results in very large bandwidth, and via Heisenberg's Uncertainty Principle, in very short pulses [5]. It turns out that prismatic arrays can be configured, at will, to either yield very high intracavity dispersion, very small intracavity dispersion, or even substract dispersion [3-6]. The linewidth equation, and the generalized prism dispersion equations, are available in PDF format. The various terms, comprising these equations, are explained in [5]. This reference also includes detailed figures describing the geometry of prismatic pulse compressors.
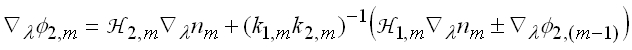
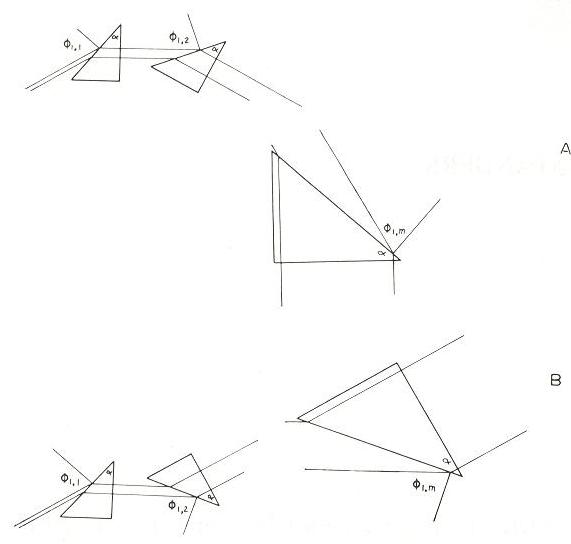
Additive, and compensating, multiple-prism arrays introduced in [3] are illustrated in Fig. 2. In the compensating configuration, shown in Fig. 2(b), the second prism substracts dispersion from the first prism. More generalized multiple-prism additive, and compensating, configurations are depicted in Fig. 3. Again, in the compensating array shown in Fig. 3 (b), the second prism substracts dispersion from the first prism.
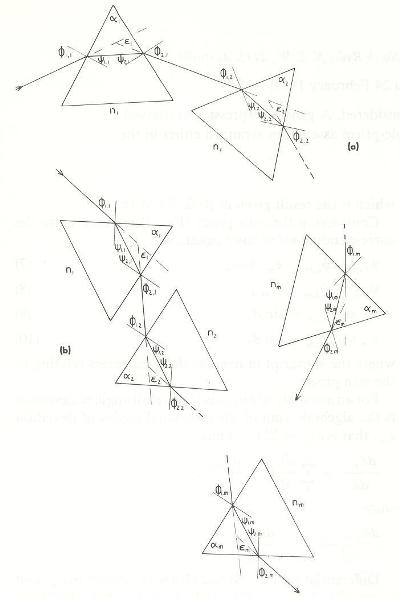
Further developments include the introduction of a fiber prism-pair pulse compressors, by Kafka and Baer [8], and the use of prismatic arrays for pulse compression in semiconductor lasers [9]. In general, the effect of minute intracavity beam deviations and geometrical perturbations on intracavity dispersion, and hence pulse compression, has been described theoretically by Duarte [10] and verified experimentally in femtosecond lasers by Osvay et al. [11]. A recent historical account of prismatic dispersion in pulse compression is given in [12] and a thorough study of dispersion control with prism pairs was performed by Arissian and Diels [13]. A survey of multiple-prism array applications is given in [14].
More on theory: multiple-prism beam expanders and arrays were described in matrix form in 1989 [15]. The multiple-prism dispersion theory was given in 4 X 4 matrix form in 1992 [16]. These matrix equations are applicable either to prism pulse compressors or multiple-pism beam expanders. These results are nicely reviewed and summarized in Tunable Laser Optics [5].
A comprehensive unified and generalized higher-order multiple-prism dispersion theory, applicable to pulse compression, is now available [17]. In this paper, generalized dispersion derivatives are given, up to the 5th order, in a mathematical framework that allows the expression of even higher derivatives, up to the Nth order, as needed. The latest reviews and updates on this subject are given in invited papers [18, 19].


Key words: pulse compresor, prism compression, prism compressor, prism compressors, prism pulse compression, prism pulse compressor, prism pulse compressors, multiple-prism compression, multiple-prism compressor, multiple-prism compressors, multiple-prism pulse compression, multiple-prism pulse compressor, multiple-prism pulse compressors.
Published on the 25th of September, 2007; updated on the 14th of January, 2020.